Section 10.2 Measures of Central Tendency and Spread
Subsection 10.2.1 Review: Mean or Average of a Discrete Random Variable
Recall that the average of a set of discrete values is the sum of the values divided by the number of values in the set. Mathematically, if there are n values in the set, and the values are denoted ai for i=1,2,…,n, then the average is aave=a1+a2+…+ann=1nn∑i=1ai. In preparation for the next section, it is helpful to consider a specific example with repeated values. Suppose that in a group of 10 people:- 5 people are 40 years old,
- 3 people are 12 years old, and
- 2 people are 7 years old.
Subsection 10.2.2 Mean or Average of a Continuous Random Variable
For the purposes of developing the formula of the mean or average of a continuous random variable, consider the probability density function f(x) for a continuous random variable X defined on the interval [a,b]. We will build a Riemann sum:- divide the interval [a,b] into n subintervals of length Δx=b−an, and
- let xi=a+i⋅Δx for i=0,1,2,…,n,
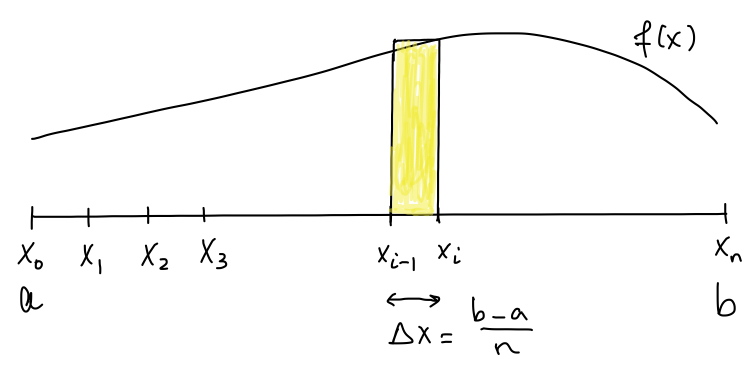
Average=x0⋅f(x0)Δx+x1⋅f(x1)Δx+…+xn⋅f(xn)Δx=n∑i=1xif(xi)Δx.
In the limit, as n→∞, and introducing the notation μ for mean or average and E[X] for expected value, we obtain μ=E[X]=∫baxf(x) dx.
In general, the mean (average) or expected value of the continuous random variable X is μ=E[X]=∫∞−∞xf(x) dx.Subsection 10.2.3 Two Additional Measures of Central Tendency: Median and Mode
The median, denoted by α, is the point at which exactly half the area under the probability density function lies to the left and the other half lies to the right, that is, the median is α such that∫∞αf(x) dx=12. The mode represents the most frequent value(s) of X. If the probability density function f(x) has a single peak, then the mode is the value of x at which f(x) has the absolute maximum. In some cases, the probability density function f(x) has multiple peaks, in which we report multiple modes, each located at a local maximum of f(x). For symmetric probability density functions with a single peak, the mean, median, and mode are the same. As we have seen, probability density functions often are not symmetric, and it is common for the mean, median, and density to be different. Each measure of central tendency has its advantages and disadvantages. One often determines all three measures of central tendency to obtain insight into the characteristics of a given continuous random variable.Remark 10.2.2.
Mean, median, and mode are not the only measures of central tendency. If you are curious about additional measures of central tendency, you may find it interesting to check out this Wikipedia page on Average.Subsection 10.2.4 Variance and Standard Deviation of a Continuous Random Variable
Variance is a measure of how much a random variable is likely to deviate from the mean. The higher the variance, the more spread out the graph of the probability and vice versa. The variance of a continuous random variable X with probability density function f(x) and mean μ is defined as the expected value of the square of the deviation of a random variable from the mean, that is,
Var[X]=E[(X−μ)2]=∫∞−∞(x−μ)2f(x) dx=∫∞−∞x2f(x) dx−μ2.
The latter two forms of the variance are equivalent; the last one generally is the easiest to work with in calculations.
The standard deviation, denoted by σ or SD[X], simply is the square root of the variance, that is, σ=SD[X]=√Var[X].
In the following video, we work through the details of determining the variance and standard deviation for a continuous random variable with a given probability density function.
Subsection 10.2.5 Summary
Measures of Central Tendency.
-
A continuous random variable X with probability density function f(x) has the following measures of central tendency:
- The mean, also known as the average or expected value, is μ=E[X]=∫∞−∞xf(x) dx.
- The median is α such that∫∞αf(x) dx=12.
- The modes are all values of x where f(x) has a local maximum.
Measures of Spread.
-
A continuous random variable X with probability density function f(x) has the following measures of spread:
- The variance isVar[X]=∫∞−∞(x−E[X])2f(x) dx=∫∞−∞(x−μ)2f(x) dx=∫∞−∞x2f(x) dx−(E[X])2=∫∞−∞x2f(x) dx−μ2.
- The standard deviation is σ=SD[X]=√Var[X].
- The variance is
Subsection 10.2.6 Don't Forget
Don't forget to return to eClass to complete the pre-class quiz.Subsection 10.2.7 Further Study
Remember that the notes presented above only serve as an introduction to the topic. Further study of the topic will be required. This includes working through the pre-class quizzes, reviewing the lecture notes, and diligently working through the homework problems. As you study, you should reflect on the following learning outcomes, and critically assess where you are on the path to achieving these learning outcomes:Learning Outcomes
- Determine the mean (a.k.a. expected value or average), median, and mode of a continuous random variable given its probability density function (PDF).
- Determine the variance and standard deviation of a continuous random variable given its probability density function (PDF).
| Learning Outcome | Video | Textbook Section |
|---|---|---|
| 1 | 10.E3 | 12.5 |
| 2 | 10.E4 | 12.5 |