After laser cooling is complete, the atoms are not quite cold enough to reach the “quantum degenerate” regime in which their behaviour is truly collective. To get their, most experiments rely on evaporative cooling, which works on the same general principles as does the cooling of a cup of tea, or our bodies through perspiration.
First, the atoms are captured either in a magnetic or optical trap. Magnetic traps work for atoms whose internal states have Zeeman energies that are higher in high magnetic fields, so that they prefer to live in low-magnetic field regions. These types of “magnetic bottles” can be created in a variety of geometries. Optical traps can work with any internal state of the atom, and rely on small energy shifts induced by second-order processes for far-off-resonant red-detuned light. In both cases, the traps are generally harmonic and the depth of the trap can be controlled.
When a cup of tea cools, it does so because the most energetic water molecules escape. The molecules which have become steam trade some of their kinetic energy to overcome the heat of evaporation, and when they do, they can leave the cup, and with it, their energy. Because only the most energetic molecules can overcome this evaporation barrier, the atoms which leave have, on average, more energy than the atoms in the cup, and the total average energy of the tea is reduced.
In the same way, we design our experiments so that the most energetic atoms will leave the system. In a trap with a finite depth, the most energetic atoms will occasionally reach the top of the trap and escape, leaving the ones behind with a lower average energy. The atoms are always colliding with each other and exchanging their energies to reach a new equilibrium. To keep up with this thermalizing reequilibration, we continuously lower the trap depth allowing the newest high energy atoms to escape. As the atoms cool and rethermalize, their density also increases, which makes reaching the quantum regime even easier. In the end, we generally keep only 0.1 to 1 % of the atoms we began with in the evaporative cooling process, but these atoms can be very cold -- generally tens to hundreds of nanoKelvin.

The final result of this process is quantum degeneracy -- a state of the system where the Heisenberg uncertainty principle is important, and the quantum mechanics of the system’s behaviour is important.
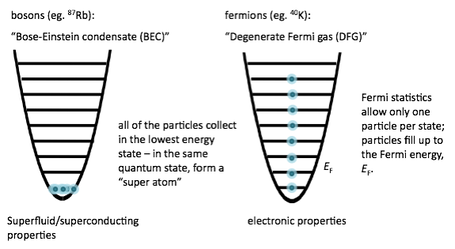
One of the quantum properties that becomes evident at this stage is the quantum statistics of the particles involved. There are two kinds of particles in the universe -- bosons and fermions -- and they behave very differently at these temperatures. All of the fundamental particles -- electrons, protons, and neutrons -- are fermions, and so, for atoms, the spin-statistics theorem says that if there is an even number of fundamental particles, the atom is a boson; if it’s odd, it’s a fermion. Among the alkalis, most stable isotopes are bosons, though two fermionic species exist -- potassium-40 and lithium-6. (The mass number hints at statistics -- the alkalis have an odd number of protons and electrons, so the number of neutrons determines the statistics. An even mass number is a fermion, an odd one a boson.)
Bosons are the “friendly” particles -- they tend to want to be together both in space, and to have as similar a momentum as possible, and so they condense, to form the Bose-Einstein condensation described earlier. The system of Bose-condensed atoms can be described as by a single wavefunction, yielding the “superatom” description mentioned before. Superfluid and superconducting systems have bosonic properties and can thus be simulated by such atomic systems. Fermions, on the other hand, are rather antisocial, as every atom in the system must occupy a different quantum state, meaning that the atoms stack up into the available energy states starting from the lowest until their are no more particles left. Electrons are fermions, and so studying their properties can lead to an understanding of the electronic properties of materials.