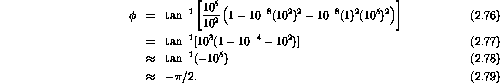Combined Impedances

We now know the impedance for each of our passive circuit elements:

The equivalent impedance of a circuit can be obtained by using the following rules for combining impedances.

In series

In parallel

Appealing to the complex notation we can write

where R is the resistance and X is called the reactance (always a function of ).

For a series combination of R, L and C

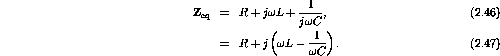
gives a special frequency, .

Example: An inductor and capacitor in parallel form the tank circuit shown in figure 2.5.

 
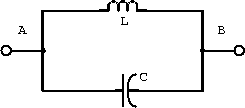
Figure 2.5:  Tank circuit with inductor and capacitor.

1. Determine an expression for the impedance of this circuit.

   The impedance of an inductor and a capacitor are

   

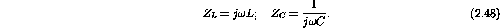
   Combining the impedances in parallel gives

   

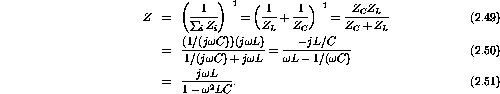
2. What is the impedance when ?

   Substituting this value for into the above result gives

   

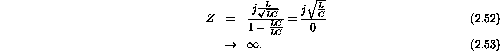
Example: The tank circuit schematic shown in figure 2.6 results from the use of a real inductor.

 
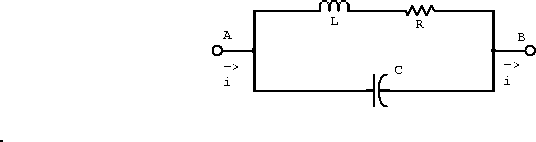
Figure 2.6:  Tank circuit with real inductor

1. Find an expression for the impedance of this circuit.

   The impedance of an inductor, capacitor and resistor are

   

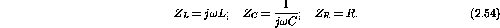
   The resistor and inductor are in series and this combination of impedance is in parallel with the capacitor. Combining the impedances gives

   

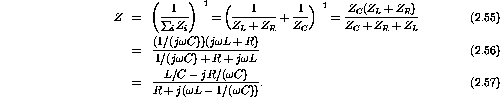
2. If L=1H, , and F, what is the impedance when ?

   Substituting this value for into the above equation gives

   

   Substituting the numerical values for the inductance, resistance and capacitance gives

   

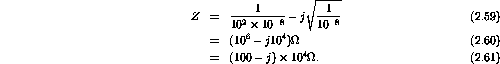
3. What is the impedance when is very small?

   

4. What is the phase angle between the voltage and at resonance and at rad/s?

   Rationalizing the denominator of the impedance gives

   

   Taking the real and imaginary components gives

   

   

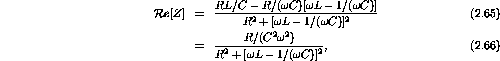
   The inverse tangent of the ratio of the imaginary to real parts is

   

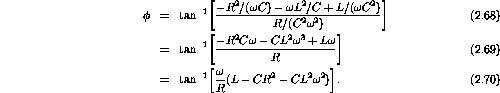
   There is a resonance at

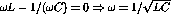
   and hence

   

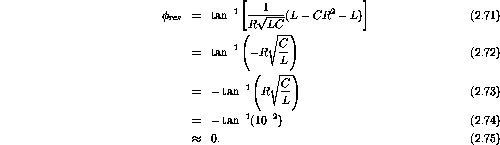
   At  rad/s.