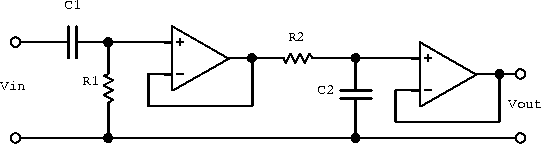
- Write an expression for the transfer function
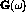 .
Express your result in terms of the amplitude of the output and
the phase relative to the input. Let
.
Express your result in terms of the amplitude of the output and
the phase relative to the input. Let 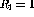 k
k  ,
,
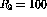
 ,
, 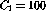
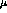 F and
F and 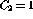
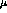 F. Do
not simplify the algebra.
F. Do
not simplify the algebra.
- What are the (real) zeroes in the transfer function, if any?
- What are the (real) poles in the transfer function if any?
- Sketch the transfer function as a function of
 on a
log-log plot. Your sketch should show the slope of
on a
log-log plot. Your sketch should show the slope of 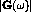 in the large and small
in the large and small  limits, the corner
frequencies, and the value of
limits, the corner
frequencies, and the value of 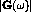 at the corner
frequencies.
at the corner
frequencies.
- Describe the dependence of the output on frequency at small and large frequencies in dB/octave.
- Write the two rules for the analysis of circuits which utilize ``ideal'' op-amps.
- Write an expression for the potential
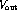 for the
following circuit.
for the
following circuit.
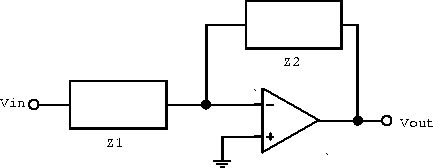
- Let
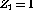 k
k  and
and 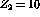 k
k  . Sketch a log-log
plot showing the function
. Sketch a log-log
plot showing the function 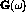 for the above
circuit assuming that a general purpose op-amp such as the 741
is used.
for the above
circuit assuming that a general purpose op-amp such as the 741
is used.
- The 741 op-amp has a corner frequencies of 4 Hz, DC open-loop
gain of
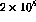 and a fall off at high frequency of
6 dB/octave. What is the frequency domain over which the
amplifier defined in part (c) will have constant gain? What is
the gain of the amplifier in this frequency domain?
and a fall off at high frequency of
6 dB/octave. What is the frequency domain over which the
amplifier defined in part (c) will have constant gain? What is
the gain of the amplifier in this frequency domain?
- Suppose now that the impedance
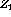 is replaced with a
capacitor with a capacitance C (
is replaced with a
capacitor with a capacitance C ( 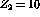 k
k  ). For
frequencies much greater than 4 Hz, the 741 op-amp will
attenuate the signal if the product RC is greater than some
maximum value. For a frequency of 50 kHz, what is the maximum
value for C for which the op-amp
). For
frequencies much greater than 4 Hz, the 741 op-amp will
attenuate the signal if the product RC is greater than some
maximum value. For a frequency of 50 kHz, what is the maximum
value for C for which the op-amp 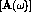 will not
attenuate the output signal at high frequencies? Hint: If
you sketch
will not
attenuate the output signal at high frequencies? Hint: If
you sketch 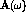 and
and 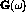 , you will be
able to see the constraint on
, you will be
able to see the constraint on 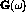 .
.
![]()
Hint: the input is ![]() .
.