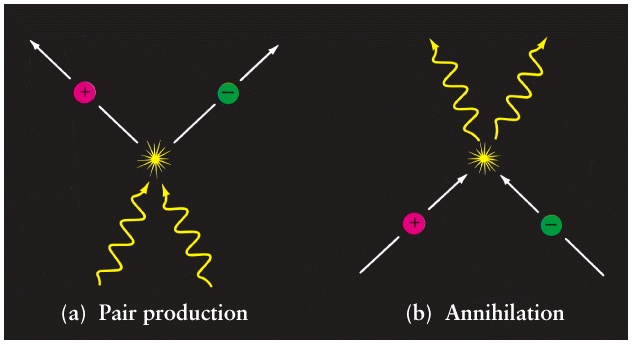
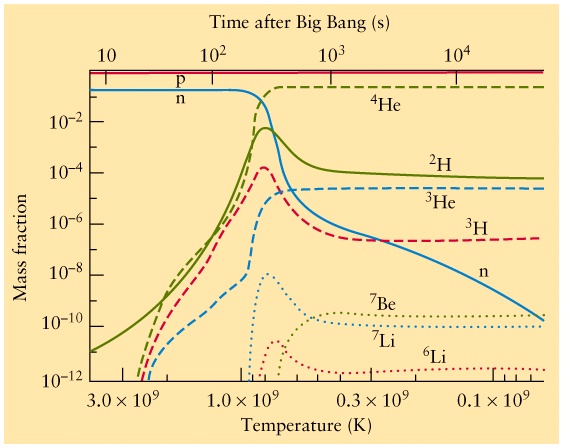
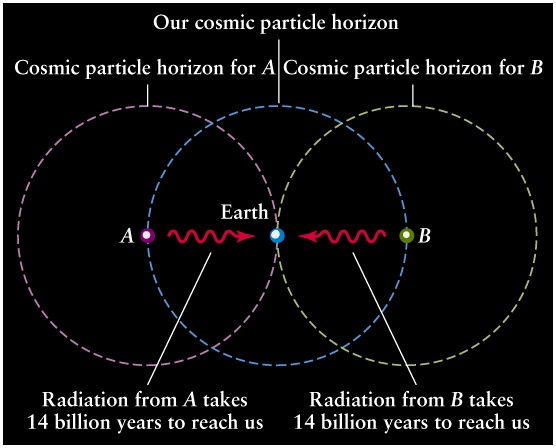
The Initial Singularity
- When the Universe was very young, the distances between
things were very tiny.
- Quantum mechanics is needed to describe very tiny distances.
- For instance Heisenberg's uncertainty relation should apply here.
- The equations describing cosmology come out of the theory
of general relativity which does not include quantum mechanics.
- The equations of general relativity are only valid when
describing distances larger than 10-35m.
- Or equivalently, general relativity only describes the
universe when it is older than 10-44 s.
- In order to describe the earliest times, a theory which
combines gravity and quantum mechanics is needed (called quantum gravity).
- One theory which might work is called Superstring Theory.
- Superstring theory predicts that the universe is actually
10 dimensional, but that we only directly experience 3 dimensions.
- Superstring theory may be able to describe the earliest times
properly.
- This is still unknown territory!
|
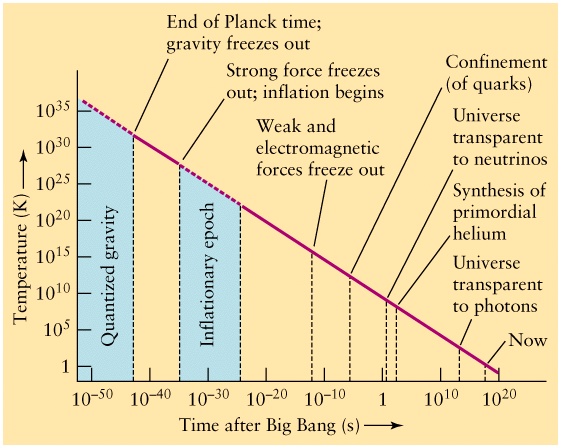 |