Lecture 20: Black Holes

The Black Holes

Black holes are the astronomical "objects" left over when the outward pressure in a star is insufficient to balance the force of gravity.
All the matter in the star is attracted to the centre of the star.
The matter collects in a region with zero volume called a singularity.
Although all the matter from the original star has condensed to the singularity, the mass of the black hole is the same as the mass of the star.
Far from a black hole, the gravitational attraction between the black hole and other objects is the same as the attraction between a regular star with the same mass as the black hole.

The most important feature of a black hole is its event horizon.

The event horizon is an imaginary surface which acts as a boundary for the black hole.

The black hole's "interior" is the region inside of the event horizon.

The black hole's "exterior" is the region outside the event horizon.

The event horizon is a sphere with a radius R_EH defined so that the escape velocity from the event horizon is exactly equal to the speed of light.

At even horison escape velocity
v = (2GM/R_EH)^1/2 = c

So for black hole of mass M, then the event horizon has a radius of
R_Sch = R_EH = 2GM/c² = 3km M/M_Sun

Since nothing can travel faster than light, it is impossible for any particles to escape from the black hole.

Light also can't escape from the black hole, hence the name.

Concepts of Space, Time and Relativity

Before the Ancient Greeks: The Earth is Flat

Earth is flat and at rest.
Events occur at the same place if no motion of objects with respect to the Earth.
Since the Earth is flat, there is a special direction called "up".

The Ancient Greeks

The Earth is a sphere, but does not move.
No special direction, since the direction of "up" now depends on your lattitude.

Galileo and Copernicus

The Earth moves.

Galilean Relativity: Must describe motion relative to the motion of other objects.

Concept of absolute spatial relations is lost.
- If an object appears to be at rest in this room, it is really moving since the Earth moves.
- If two events happen at the same place for one observer, they will happen in different places for moving observer

Still have a concept of absolute time.

If two cars move with velocities v and V then the first driver sees the other car moving with velocity V-v

Special Relativity

It has been known since the end of 17 century (Ole Romer observations of delay in Jupitor moon Lo eclipses) that light travels and finite speed

Galileo principle: If light is emitted by an object moving with velocity v, the light will move with velocity v + c.

The Michelson-Morley Experiment

In 1887 two physicists, Michelson and Morley attempted to measure the change in the speed of light due to the motion of the emitter.

In their experiment, they measured the difference between the speed of light in the direction of the Earth's velocity and the speed of light in a direction perpendicular to the Earth's velocity.

According to Galilean relativity, these velocities should be different.

Michelson and Morley found that the speed of light was independent of the motion of the object emitting the light!

Since observers moving with different velocities must agree on the speed of light, they can't agree on the lengths of objects or the duration of events.

Einstein's Relativity

1905 - Einstein introduced the principle of special relativity.

Einstein's postulated two special relativity principles
The speed of light in vacuum will be measured to be the same, no matter how fast you move.

Main conclusions:
- No massive particle can travel faster than light.
- Observed flow of time depends on the relative motion of the clock and observer. Time dilation: Moving clock runs slower than identical clock at rest.
- Whether two events are simultaneous is not absolute and depends on observer. But for two events where one caused the other, the cause is always before the effect .
- Length contraction: Moving objects are shorter than when they are at rest.

Instead of talking separately of space and time, we talk about combinations of the two ideas called spacetime.

Einstein's General Theory of Relativity --- A Relativistic Theory of Gravity

The speed of light in vacuum is constant and is the upper speed limit.
The speed of light is maximum speed at which information can travel.

It is then clean, there is a problem with Newton's law of gravitation:
- Suppose that the Sun is suddenly moved.
- The photons from the Sun take 8 minutes to travel to the Earth, so after 8 minutes we would see that the Sun moved.
- In Newton's theory of gravity, we would feel the change in the gravity instantaneously.

Einstein realized that it should also take 8 minutes for us to feel the change in the gravity of the Sun.

1917 - Einstein introduced the general theory of relativity in order to properly explain how gravity behaves.

One prediction of Einstein's theory is that gravitational radiation transmits information about the change in a gravitational field.

Curvature of Spacetime

Main concept of general relativity:
Gravity is the curvature of space-time.

In Einstein's theory of general relativity, we picture a massive object's gravitational field causing a rubber mat to become deformed.

Theory says that the mass (and energy) of objects causes a curvature of spacetime.

Light and other objects must follow the shortest possible path in the spacetime.

Example: Fly from Edmonton to London. The shortest path is a great circle. But it is not a straight line !

Light rays or massive particles will move on the shortest possible path on this deformed mat.

Important note: It is not just space but the whole space-time that is curved

The Einstein Equivalence Principle

One of the basic concepts in general relativity is the equivalence between gravity and acceleration.
Astronaut in a windowless rocket can't tell the difference between:

Rocket sitting at rest on the surface of the Earth.	Rocket far from any massive bodies moving with a constant acceleration = g.

All experiments done in the two rockets yield the same results.

From equivalence principle - gravity is not felt if the object is in free fall.

Why is this important ? Establishes relation between gravity and acceleration. But acceleration appears when the motion in space time is along curved paths. So link between gravity and curved geometry of space-time is incorporated in this principle.

Here is for example how one can see from equivalence principle that light must bend in the presence of gravity.

Elevator free-falls. There is no gravity felt inside, special relativity holds, light propagates along the straight line.

For the observer on Earth, that feels gravity, the light must follow the curve path !, So gravity bends the light rays.

Bending of Light

In order for light to travel at the same speed, photons have to move on curved paths when a massive object is present.

We call this effect the gravitational bending of light.

This effect was first seen during a solar eclipse in 1919.

A star which should have been directly behind the Sun was visible, because the star's photons moved on a curved path around the Sun.

Perihelion of Mercury

The Sun's gravity is explained very well with Newton's theory of gravity.

However, close to the Sun, Newton's laws aren't exactly correct and Einstein's theory of gravity must be used.

Under Newton's theory, the orbit of the closest planet, Mercury, should be exactly an ellipse which retraces the same path.

Under Einstein's theory, the orbit is almost an ellipse, but the orbit doesn't close in on itself, and a flower pattern is traced out over time. (Technical name = "Perihelion Precession")

Mercury's orbit follows exactly the flower pattern predicted by Einstein.

Note: the effect in this diagram is greatly exagerated!

Gravitational Redshift

Einstein's theory of gravity also predicts that when light is emitted from the surface of a planet or a star, its wavelength gets redshifted as it moves away from the star.

Equivalent to say that clocks tick slower in a strong gravitational field compared to clocks far from the gravitational field. This is called gravitational time dilation . This is not Doppler effect due to motion of the source !

This effect is tiny for the Earth ( ~10^-15 but was measured in 1960 !) , but it must be taken into account in the GPS satellite system used for finding positions on the Earth!

This redshift has also been observed from light emitted from the surface of white dwarfs and neutron stars. It is of order ~10^-4 for white dwarfs and ~0.1 for neutron stars.

Properties of Black Holes

Dark Star

The concept of a black hole (or dark star) was introduced in the late 1700's by Michell and Laplace.

They considered a very dense star with an escape velocity greater than light.

This star would appear black since no light could escape from its surface.

Concept of a Black Hole in Relativity

The material inside of the dark star can't be at rest. It all falls into the singularity at r=0.

The event horizon is at the same location as the surface of the dark star conjectured by Michell and Laplace.

Inside of the event horizon, a massive particle or a photon would have to move faster than light to get outside of the black hole.

Since nothing travels faster than light, you can't see the inside of a black hole.

If you are outside the black hole, the gravity is just like the gravity of a star with the same mass as the black hole.

If our Sun were suddenly replaced by a black hole with Mass = M_Sun, the orbits of the planets wouldn't change.

If you travelled close to the black hole, you could escape, as long as you don't enter the event horizon.

If you entered the black hole, you could still see everything outside, since light can enter a black hole.

You wouldn't notice anything special about the event horizon, since it isn't a solid surface.

Once you enter the event horizon, you will pulled into the singularity.

A trip into a black hole -- Tides

A trip into a black hole would probably be fatal.

Tides are created when the force of gravity is stronger on one side of an object than on the other.

If you were falling feet first into a black hole, the force of gravity acting on your feet would be stronger than the force of gravity acting on your head.

The effective tidal force would stretch you lengthwise and compress your width.

A trip into a black hole -- Gravitational Redshift and Time Dilation

Suppose that a rocket decided to enter a black hole.	The rocket sends a burst of light every second back towards Earth where we watch the signals.
The gravitational time dilation effect means that the interval between our detection of the light bursts gets longer as the rocket gets closer to the black hole.	The photon's wavelength gets redshifted, so that if the rocket emits blue light, we will detect some redder colour.

On our clock, it takes an infinite amount of time for the rocket to reach the event horizon.	But the astronauts in the rocket think that the trip only takes a short, finite amount of time.

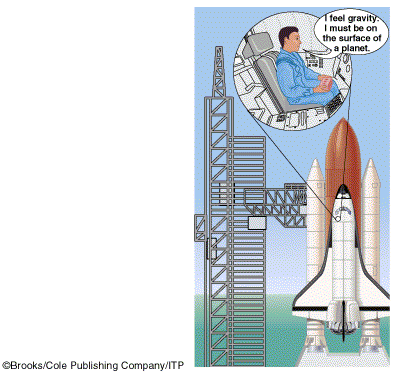
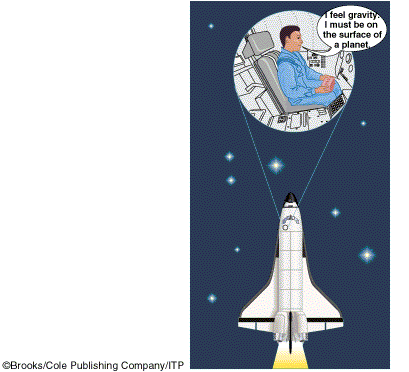
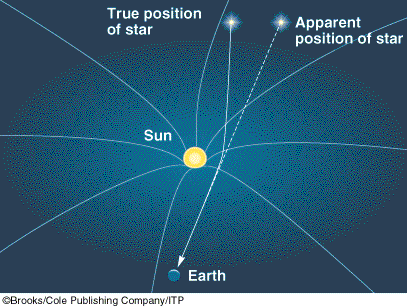
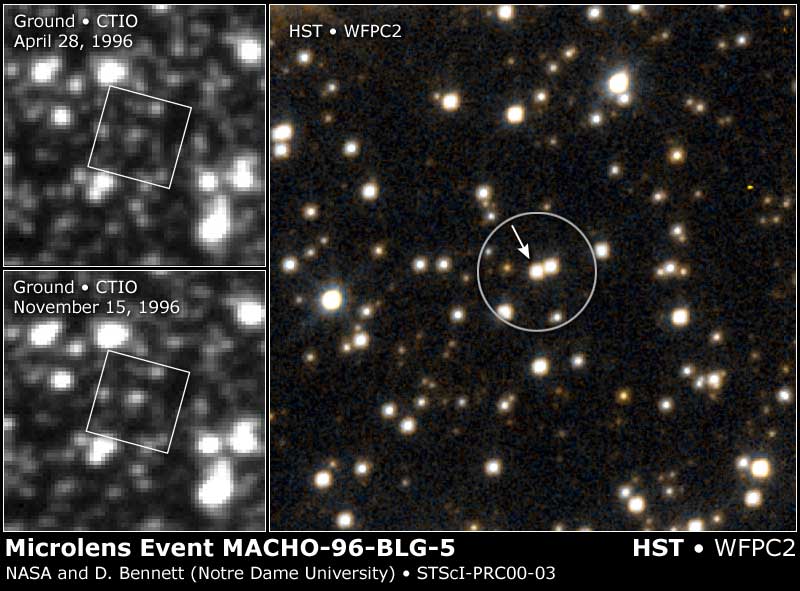
Black holes are simple!

Although a star is approximately a sphere, it also has many bumps (ie. prominences, flares, etc).

As the density of a star increases, the size of bumps has to decrease, ie. gravity smooths out bumps.

For instance, mountains on a neutron star can't be much higher than a centimetre.

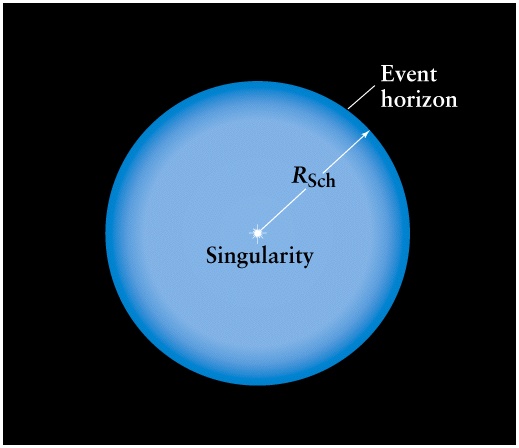
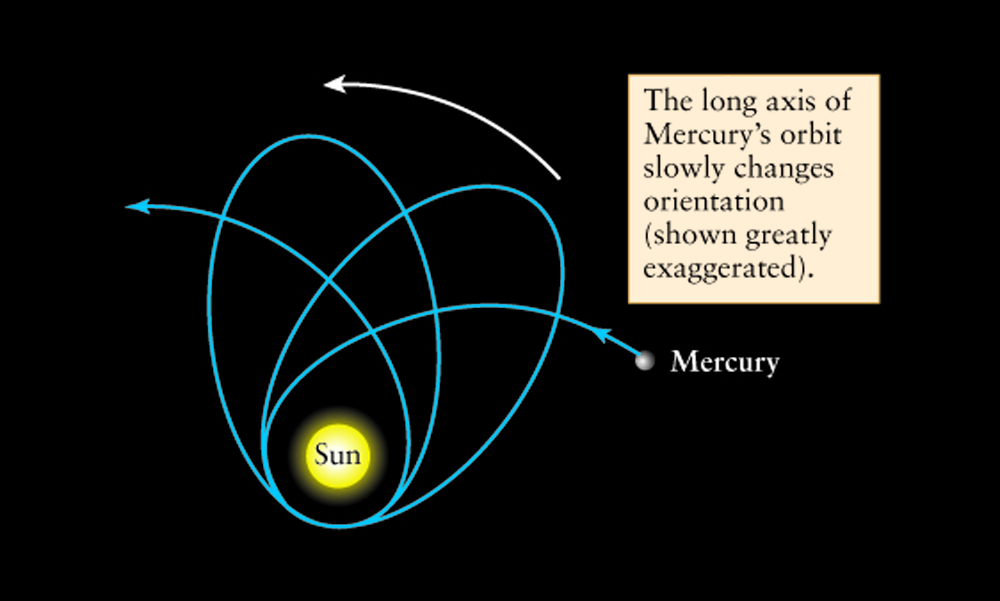
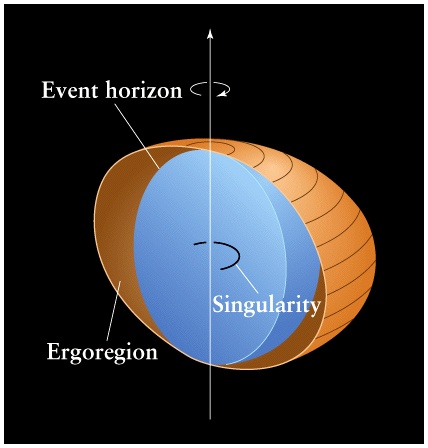
In 1967, Werner Israel, (professor at the U of A, now retired in Victoria) proved the "No Hair" Theorem which states that the event horizon has to be perfectly smooth.

From Israel's theorem, a black hole's properties are given by just three numbers &minus mass M, electric charge Q and angular momentum L.

If the black hole doesn't rotate, it must be exactly spherical.

If the black hole rotates, it must have a special ellipsoidal shape.

Black Holes Evaporate

In quantum mechanics, a vacuum really isn't a vacuum.
Pairs of particles and anti-particles are constantly being created and destroyed.
The higher the mass of the particles, the shorter the time they exist, through Heisenberg's uncertainty principle:
mass x c² x time = h/(2 &pi)

Hawking Radiation

1974 - Stephen Hawking showed that if pairs are created near the event horizon, it is possible for them to be separated so that one falls into the black hole and one escapes.
Far from the black hole, an observer would see a flux of massive particles coming from the black hole.
The energy allowing this flux is coming from the mass of the black hole itself, so the black hole must lose mass.
The flux obeys the Stefan-Boltzmann law, so a temperature can be assigned to the black hole.
This temperature is inversely proportional to the black hole's mass.

Consider two masses:
A black hole with M = 5 M_Sun
- T = 10^-7 K, which isn't measureable
- This black hole would take 10⁶² years to evaporate!
A black hole with M = 10¹⁰ kg = 10^-20 M_Sun
- T = 10¹⁴ K
- This black hole would take 15 x 10⁹ years to evaporate!
- But such small mass black holes can't be formed from the cores of stars.
- Tiny black holes could have been created in the early universe, but no such evidence yet.

How do you detect an invisible object?

Black holes are detected by observing their gravitational effect on other objects.

Stellar mass black holes

Binary systems including a black hole

Consider a binary system consisting of a regular star and an invisible star.
By observing the orbit of the regular star, you can find the mass of the invisible star, if you know the angle of inclination of the binary.
i = angle of inclination = angle between the plane of the binary's orbit and the plane of the sky.
If angle is unknown you can only find a lower limit on the mass of the invisible star.
Neutron stars are very dim and could appear to be invisible.
If the minimum mass measured is larger than the maximum mass for a neutron star (about 3 or 4 times the mass of the Sun), the invisible star is a black hole.
Since this is an indirect method for finding a black hole, the objects found this way are called Black Hole Candidates.


	The companion star to Cyg X1

Accretion onto a Black Hole or a Neutron Star

When gas falls towards a star, gravitational potential energy is converted to kinetic energy.
The total energy of the gas is E = K - GM/r
K = kinetic energy
Energy is conserved as the gas falls.
Suppose that the gas has zero energy, then K = GM/r
When the gas is far from the star, the Kinetic energy is small.
When the gas falls closer to the star, the Kinetic energy increases.
The kinetic energy of a gas is proportional to its temperature.
The gas will radiate as it falls, and the higher the Kinetic energy, the higher energy will the photons radiated be.
Neutron stars and Black holes have such small sizes, that 1/r is very large.
The gas falling into either a Neutron star or a Black Hole radiates X-rays.
A binary system which emits X-rays is called an X-ray Binary.


	X-ray Picture of the LMC X1 Black Hole Candidate

Differences Between Accreting Neutron Stars and Black Holes

All of the Black hole candidates listed above are members of X-ray binaries.

One important difference between neutron stars and black holes:
- Black holes do not have a hard surface.
- When Hydrogen falls into the black hole, there is no surface on which nuclear explosions can take place.
- If X-ray bursts from nuclear explosions take place, the star must be a neutron star, not a black hole.
- (Unfortunately, the converse is not true.)

It is also possible for gas in the disk to flare. The X-rays from X-ray binaries tend to vary rapidly with time.

Since gas is lost when it falls into the event horizon, the disks surrounding black holes should be a little dimmer than neutron stars. Some evidence that this signature of less light has been observed.

Supermassive (~ 10⁶ M_Sun) Black Holes Black Holes at the Centres of Galaxies At the centres of galaxies supermassive black holes can form. These black holes also have accretion disks which emit x-rays. More about the black holes in the centres of galaxies when we study galaxies.
One of the best black hole candidates- supermassive (10⁶ M_Sun) black hole at the center of our Galaxy	Stars Orbiting Black Hole at Center of Milky Way Loose Årrow \| MySpace Video

Other Places Where Black Holes Could Be Found:

Supernova Remnants

Black holes are supposed to be formed in supernovae.
However, no conclusive evidence has been found yet for a black hole found inside of a supernova remnant.
However, some of the supernova remnants do not have evidence for a neutron star, so perhaps a black hole is in them.

Gamma-Ray Bursts

The latest theories of gamma-ray bursts (which we will study later on in a couple of weeks) suggests that they occur when a rapidly rotating black hole is formed in a severe type of supernova.

Gravitational Lensing

The gravitational field of any mass curves space so that light travels on a curved path.
If a massive object (a black hole, for instance) is in front of a star, we can still see the light from the star since the light moves around the massive object.
We call this effect gravitational lensing since a mass acts like a lens.

Another effect due to gravitational lensing is that the star being lensed by the foreground mass gets brighter when the mass passes in front of the star.
It is possible to measure the mass of the "lens".
A few black holes have been detected as follows:
- A black hole passes in front of a background star.
- (The black hole is closer to us, so it moves faster than the star.)
- The star appears to be brighter when the black hole passes in front.
- An important fact about the lensing: Both blue and red light get brighter by the same amount.
- If the star just happened to brighten on its own, the blue and red light would not increase at the same rate.

Next lecture: The Milky Way Galaxy