
Lesson 4: Whispering gallery modes
lesson 1 lesson 2 lesson 3 lesson 5
So now we get to the really fun stuff. Just like for acoustic waves, we can make "whispering galleries" for light waves. But we have to worry about two additional things. First, we need good reflective surfaces for light to "bounce" from - just like sound bouncing off the walls of St. Paul's. Second, to get analogous effects, we need our gallery to be much smaller than a real cathedral. This is because visible light waves are about 10,000,000 times smaller than audible sound waves, give or take.
You might think that a metal mirror like the one in your bathroom might be a good reflective surface, but it actually isn't nearly good enough. Common silver household mirrors reflect about 97% of the light energy that hits them. But for a gallery to work we need to have many, many reflections as the waves bounce around the gallery. If we lost 3% each time we'd soon have nothing left!
Luckily, it is possible to solve the chamber size and reflectivity problem in one fell swoop. An example of an optical gallery from our lab is shown below:
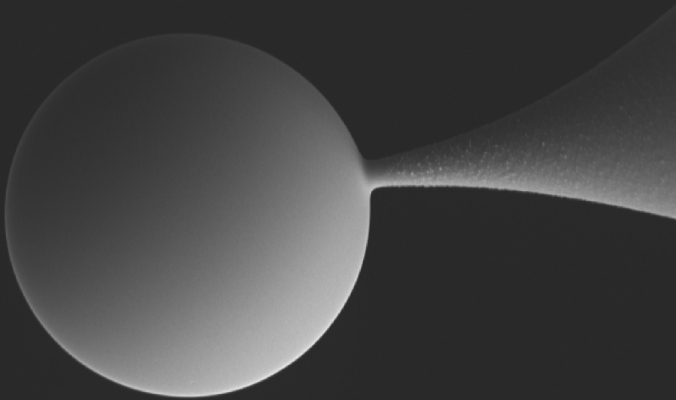
The image is of a glass microsphere sitting on the end of a tapered optical fiber. It's about half the diameter of a typical human hair, and about ten million times smaller than St. Paul's cathedral. If we can get light inside the glass sphere, it gets trapped by a phenomenon called "total internal reflection". This is basically a property of light that, when it hits an interface between a material of high index of refraction (i.e., an optically dense material like glass) and a lower one like air, it can get reflected perfectly.
This property is actually the basis of how fiber optics work - it's why we can send light pulses down glass fibers that are hundreds of km long. In fact, if it weren't for total internal reflection you couldn't be reading this web page.
So we get light bouncing around this tiny gallery. Just like for sound, flat membranes, and strings, we get resonances at certain specific frequencies.
So how do we observe these optical whispering gallery modes? In our lab, we coat microsphere or microcylinder "galleries" with a fluorescent material known as silicon quantum dots. Fluorescent materials emit light when excited with other light - for us the fluorescence gets trapped in the gallery and begins to resonate.
Look at the fluorescence spectra below: the sharp peaks are the optical WGMs of a sphere like the one above. To the eye the color of this spectrum looks deep red, but the emitted light actually consists of a set of pure, narrowly-spaced wavelengths that represent the optical resonances. The two spectra are labeled "TE" and "TM", and they represent two different polarizations that are resonating in the gallery.

One of the fun things is that the electric field associated with optical resonances can be calculated pretty easily. The derivation of the field comes straightforwardly from the Helmholtz equation. It take some messing around with Bessel functions, as one would expect for cylindrical or spherical resonances, but good math software can really make this job easy. We like Mathematica especially for many reasons, and students in our group all get trained up in how to do basic coding. Once you have that down, it's easy to make beautiful plots of the electric field going around the microcavity.
Here, we can see an part of an optical resonance modeled using equations. In this case the light is traveling counterclockwise. If we added a clockwise-traveling beam of the same wavelength, we'd get a perfect resonance like the ones shown on the next page. The particular resonance shown below is for a layered circular structure, similar to the materials we make in the lab.

On to lesson 5. Warning: it gets a bit more technical!